Category: Machine Learning

New open-source library: Conditional Gaussian Mixture Models (CGMM)

August 31, 2025 / Thijs van den Berg

pip install cgmm

I’ve released a small, lightweight Python library that learns conditional distributions and turns them e.g. into scenarios, fan charts, and risk bands with just a few lines of code. It’s built on top of scikit-learn (fits naturally into sklearn-style workflows and tooling).

Example usage:

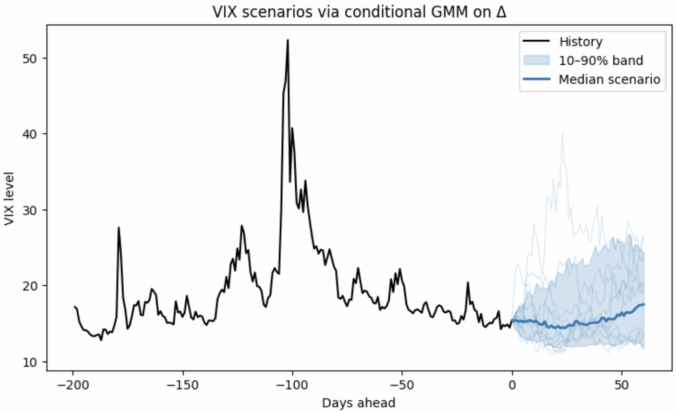
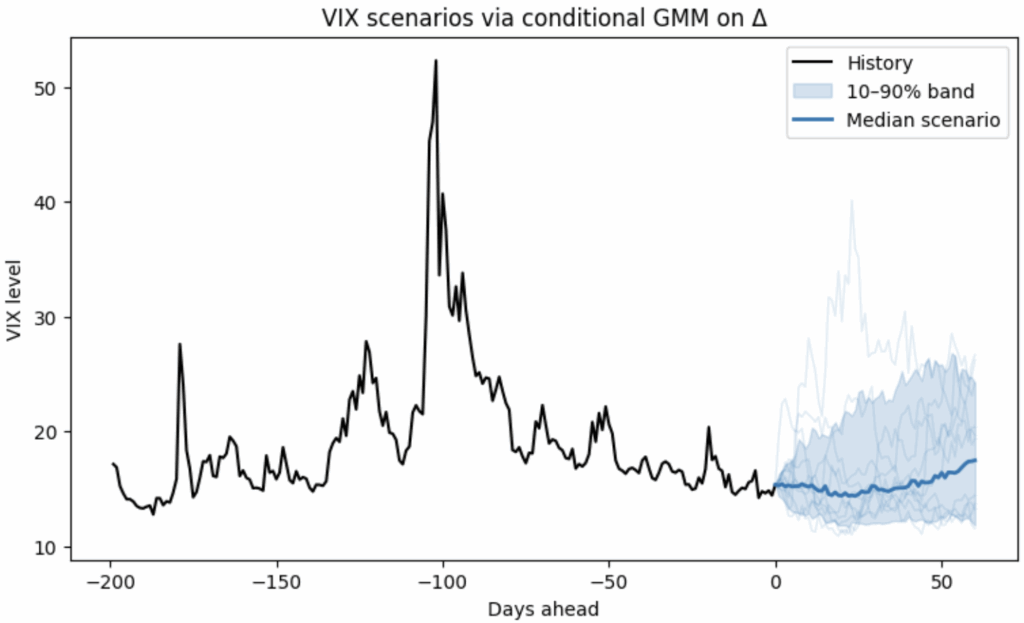
In the figure below, a non-parametric model is fit on ΔVIX conditioned on the VIX level, so it naturally handles:

Non-Gaussian changes (fat tails / asymmetry), and
Non-linear, level-dependent drift (behavior differs when VIX is low vs. high).

Features:

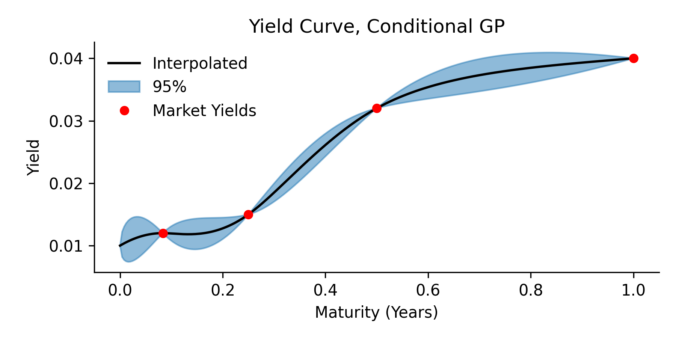
Conditional densities and scenario generation for time series and tabular problems
Quantiles, prediction intervals, and median/mean paths vuia MC simulation
Multiple conditioning features (macro, technicals, regimes, etc.)
Lightweight & sklearn-friendly; open-source and free to use (BSD-3)

VIX example notebook: https://cgmm.readthedocs.io/en/latest/examples/vix_predictor.html

Call for examples & contributions:

Do you have a use-case we should showcase (rates, spreads, realized vol, token flows, energy, demand, order-book features…)?
Send a brief description or PR—examples will be attributed.
Contributions, issues, and feature requests are very welcome. And if you find this useful, please share or star the repo to help others discover it.

Not investment advice. The library is still work in progress!

Yield Curve Interpolation with Gaussian Processes: A Probabilistic Perspective

March 23, 2025 / Thijs van den Berg

Here we present a yield curve interpolation method, one that’s based on conditioning a stochastic model on a set of market yields. The concept is closely related to a Brownian bridge where you generate scenario according to an SDE, but with the extra condition that the start and end of the scenario’s must have certain values. In this paper we use Gaussian process regression to generalization the Brownian bridge and allows for more complicated conditions. As an example, we condition the Vasicek spot interest rate model on a set of yield constraints and provide an analytical solution.

The resulting model can be applied in several areas:

Monte Carlo scenario generation
Yield curve interpolation
Estimating optimal hedges, and the associated risk for non tradable products

Finding the Nearest Valid Correlation Matrix with Higham’s Algorithm

March 12, 2025 / Thijs van den Berg

Introduction

In quantitative finance, correlation matrices are essential for portfolio optimization, risk management, and asset allocation. However, real-world data often results in correlation matrices that are invalid due to various issues:

Merging Non-Overlapping Datasets: If correlations are estimated separately for different periods or asset subsets and then stitched together, the resulting matrix may lose its positive semidefiniteness.
Manual Adjustments: Risk/assert managers sometimes override statistical estimates based on qualitative insights, inadvertently making the matrix inconsistent.
Numerical Precision Issues: Finite sample sizes or noise in financial data can lead to small negative eigenvalues, making the matrix slightly non-positive semidefinite.

Optimal Labeling in Trading: Bridging the Gap Between Supervised and Reinforcement Learning

March 10, 2025 / Thijs van den Berg

When building trading strategies, a crucial decision is how to translate market information into trading actions.

Traditional supervised learning approaches tackle this by predicting price movements directly, essentially guessing if the price will move up or down.

Typically, we decide on labels in supervised learning by asking something like: “Will the price rise next week?” or “Will it increase more than 2% over the next few days?” While these are intuitive choices, they often seem arbitrarily tweaked and overlook the real implications on trading strategies. Choices like these silently influence trading frequency, transaction costs, risk exposure, and strategy performance, without clearly tying these outcomes to specific label modeling decisions. There’s a gap here between the supervised learning stage (forecasting) and the actual trading decisions, which resemble reinforcement learning actions.

In this post, I present a straightforward yet rigorous solution that bridges this gap, by formulating label selection itself as an optimization problem. Instead of guessing or relying on intuition, labels are derived from explicitly optimizing a defined trading performance objective -like returns or Sharpe ratio- while respecting realistic constraints such as transaction costs or position limits. The result is labeling that is no longer arbitrary, but transparently optimal and directly tied to trading performance.

Parameter Grid-searching with Python’s itertools

December 29, 2020 / Thijs van den Berg

Python’s Itertools offers a great solution when you want to do a grid-search for optimal hyperparameter values, -or in general generate sets of experiments-.

In the code fragment below we generate experiment settings (key-value pairs stored in dictionaries) for all combinations of batch sizes and learning rates.

import itertools

# General settings
base_settings = {'epochs': 10}

# Grid search
grid = {
    'batch_size': [32, 64, 128],
    'learning_rate': [1E-4, 1E-3, 1E-2]
}

# Loop over al grid search combinations
for values in itertools.product(*grid.values()):
    point = dict(zip(grid.keys(), values))

    # merge the general settings
    settings = {**base_settings, **point}

    print(settings)

output:

{'epochs': 10, 'batch_size': 32, 'learning_rate': 0.0001}
{'epochs': 10, 'batch_size': 32, 'learning_rate': 0.001}
{'epochs': 10, 'batch_size': 32, 'learning_rate': 0.01}
{'epochs': 10, 'batch_size': 64, 'learning_rate': 0.0001}
{'epochs': 10, 'batch_size': 64, 'learning_rate': 0.001}
{'epochs': 10, 'batch_size': 64, 'learning_rate': 0.01}
{'epochs': 10, 'batch_size': 128, 'learning_rate': 0.0001}
{'epochs': 10, 'batch_size': 128, 'learning_rate': 0.001}
{'epochs': 10, 'batch_size': 128, 'learning_rate': 0.01}